Question

In a poll of 745 randomly selected adults, 591 said that it is morally wrong to not report all income on tax returns. Use a 0.01 significance level to test the claim that 75% of adults say that it is morally wrong to not report all income on tax returns.

Answer 1

The calculated value Z = 2.734 > 2.576 at 0.01 level of significance

Null hypothesis is rejected

The claim that 75% of adults say that it is morally wrong to report all income on tax returns.

Explanation:

Step(i):-

Given random sample size 'n' = 745

Given In a poll of 745 randomly selected adults, 591 said that it is morally wrong to not report all income on tax returns

sample proportion

`p^(-) = (x)/(n) = (591)/(745) = 0.7932`

Given Population proportion P = 0.75

Q = 1-P

Q = 1 - 0.75 = 0.25

Null Hypothesis :H₀: P=0.75

Alternative Hypothesis :H₁:P≠ 0.75

Step(ii):-

Test statistic

`Z = \frac{p^(-)-P }{\sqrt{(P Q)/(n) } }`

`Z = \frac{0.7932-0.75 }{\sqrt{(0.75 X 0.25)/(745) } }`

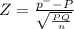
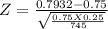
Z = 2.734

Level of significance ∝ = 0.01

The critical value Z =2.576

The calculated value Z = 2.734 > 2.576 at 0.01 level of significance

Null hypothesis is rejected

The claim that 75% of adults say that it is morally wrong to report all income on tax returns.