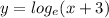Question

Determine the function which corresponds to the given graph. (3 points) a natural logarithmic function crossing the x axis at negative two and y axis at one.

The asymptote is x = -3.

Answer 1

`y =log_e(x+3)`

Explanation:

It is given that the graph corresponds to a natural logarithmic function.

That means, the function
`y` has a natural log (Log with base
`e`) of some terms of x.

It is given that asymptote of given curve is at
`x= -3`. i.e. when we put value

`x= -3`, the function will have a value
`y \rightarrow \infty`.

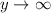We know that natural log of 0 is not defined.

So, we can say the following:

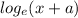`log_e(x+a)` is not defined at
`x= -3`

`\Rightarrow x+a =0\\\Rightarrow x = -a`

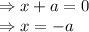
i.e.
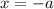`x =-a` is the point where
`y \rightarrow \infty`

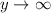
a = 3

Hence, the function becomes:

`y =log_e(x+3)`

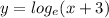
Also, given that the graph crosses x axis at x = -2

When we put x = -2 in the function:

`y =log_e(-2+3) = log_e(1) = 0`

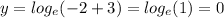
And y axis at 1.

Put x = 0, we should get y = 1

`y =log_e(0+3) = log_e(3) \approx 1`

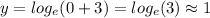
So, the function is:
`y =log_e(x+3)`