Question

PLEASE HELP.........

The graph of which of the following inequalities has open circles on -8 and 2 with a line segment between them?

A. Ix +3I < -5
B. Ix + 8I < 2
C. Ix + 3I < 5​

Answer 1

Answer: Choice C. |x+3| < 5

=======================================================

Step-by-step explanation:

Let's go through each answer choice and solve for x

----------------------------------------------------------------------

Choice A

|x+3| < -5

This has no solutions because |x+3| is never negative. It is either 0 or positive. Therefore, it can never be smaller than -5. So we can rule this out right away.

----------------------------------------------------------------------

Choice B

|x+8| < 2

-2 < x+8 < 2 .... see note below

-2-8 < x+8-8 < 2-8 ... subtract 8 from all sides

-10 < x < -6

We will have a graph where the open circles are at -10 and -6, with shading in between. This does not fit the original description. So we can rule this out too.

----------------------------------------------------------------------

Choice C

|x+3| < 5

-5 < x+3 < 5 .... see note below

-5-3 < x+3-3 < 5-3 .... subtracting 3 from all sides to isolate x

-8 < x < 2

We found our match. This graph has open circles at -8 and 2, with shading in between. The open circles indicate to the reader "do not include this value as a solution".

----------------------------------------------------------------------

note: For choices B and C I used the rule that
`|x| < k` turns into
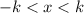`-k < x < k` where k is some positive number. For choice A, we have k = -5 which is negative so this formula would not apply.