Answer:
A. Margin of error = 128.79
B. The 99% confidence interval for the population mean is (7661.21, 7918.79).
Explanation:
We have to calculate a 99% confidence interval for the mean.
The population standard deviation is know and is σ=500.
The sample mean is M=7790.
The sample size is N=100.
As σ is known, the standard error of the mean (σM) is calculated as:
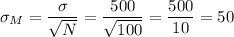
The z-value for a 99% confidence interval is z=2.576.
The margin of error (MOE) can be calculated as:
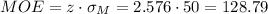
Then, the lower and upper bounds of the confidence interval are:
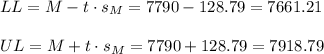
The 99% confidence interval for the population mean is (7661.21, 7918.79).