Question

In the game of​ roulette, a player can place a ​$9 bet on the number 26 and have a StartFraction 1 Over 38 EndFraction probability of winning. If the metal ball lands on 26​, the player gets to keep the ​$9 paid to play the game and the player is awarded an additional ​$315. ​Otherwise, the player is awarded nothing and the casino takes the​ player's ​$9. What is the expected value of the game to the​ player? If you played the game 1000​ times, how much would you expect to​ lose? Note that the expected value is the​ amount, on​ average, one would expect to gain or lose each game.

Answer 1

(a)-$0.47

(b)$4700

Explanation:

1. If the player wins, he gets a profit of $315. The probability of winning = 1/38
2. If the player losses, he gets a profit of -$9 ( a loss of $9). The probability of losing = 37/38

(a)Therefore:

The expected value of the Game to the Player

`=((1)/(38)* \$315)+((37)/(38)* -\$9)\\=-\$0.47`

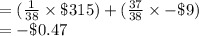
The expected value of the game to the​ player is -$0.47. This means the player is expected to lose $0.47.

(b)If the game is played 1000 times

Expected Loss = 1000 X $0.47

=$4700

Therefore, If you played the game 1000​ times, you would be expected to lose approximately $4700.