Question

What is the numerator of the simplified sum?

StartFraction x Over x squared + 3 x + 2 EndFraction + StartFraction 3 Over x + 1 EndFraction

Answer 1

C - 4x+6

Step-by-step explanation:

Edg2020

Answer 2

4x + 6

Step-by-step explanation:

`(x)/(x² + 3x + 2) + (3)/(x + 1)`

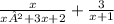
To determine what the numerator would be, after simplifying both fractions, take the following steps:

Step 1: Factorise the denominator of the first fraction, x² + 3x + 2.

Thus,

x² + 2x + x + 2

(x² + 2x) + (x + 2)

x(x + 2) +1(x + 2)

(x + 1)(x + 2)

We would now have the following as our new fractions to add together and simplify:

`(x)/((x + 1)(x + 2)) + (3)/(x + 1)`

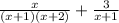
Step 2: find the highest common factor of the denominator of both fractions.

Highest common factor of (x + 1)(x + 2) and (x + 1) = (x + 1)(x + 2)

Step 3: To add both fractions, divide the highest common factor gotten in step 2 by each denominator, and then multiply the result by the numerator of each fraction.

Thus,

`(x)/((x + 1)(x + 2)) + (3)/(x + 1)`

`(x + 3(x + 2))/((x + 1)(x + 2))`

`(x + 3x + 6))/((x + 1)(x + 2))`

`(4x + 6))/((x + 1)(x + 2))`

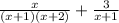
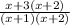
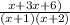
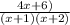
Therefore, the numerator of the simplified form sum of both fractions = 4x + 6