Question

Suppose the lengths of the pregnancies of a certain animal are approximately normally distributed with mean mu equals 247 days and standard deviation sigma equals 16 days. Complete parts​ (a) through​ (f) below.

Answer 1

The answer is given below

Explanation:

a) What is the probability that a randomly selected pregnancy lasts less than 242 days

First we have to calculate the z score. The z score is used to determine the measure of standard deviation by which the raw score is above or below the mean. It is given by:

`z=(x-\mu)/(\sigma)`

Given that Mean (μ) = 247 and standard deviation (σ) = 16 days. For x < 242 days,

`z=(x-\mu)/(\sigma)=(242-247)/(16)=-0.31`

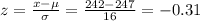
From the normal distribution table, P(x < 242) = P(z < -0.3125) = 0.3783

(b) Suppose a random sample of 17 pregnancies is obtained. Describe the sampling distribution of the sample mean length of pregnancies.

If a sample of 17 pregnancies is obtained, the new mean
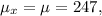`\mu_x=\mu=247,` the new standard deviation:
`\sigma_x=\sigma/√(n) =16/√(17) =3.88`

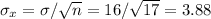
c) What is the probability that a random sample of 17 pregnancies has a mean gestation period of 242 days or less

`z=(x-\mu)/(\sigma/√(n) )=(242-247)/(16/√(17) )=-1.29`

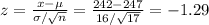
From the normal distribution table, P(x < 242) = P(z < -1.29) = 0.0985

d) What is the probability that a random sample of 49 pregnancies has a mean gestation period of 242 days or less?

`z=(x-\mu)/(\sigma/√(n) )=(242-247)/(16/√(49) )=-2.19`

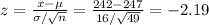
From the normal distribution table, P(x < 242) = P(z < -2.19) = 0.0143

(e) What might you conclude if a random sample of 49 pregnancies resulted in a mean gestation period of 242 days or less?

It would be unusual if it came from mean of 247 days

f) What is the probability a random sample of size 2020 will have a mean gestation period within 11 days of the mean

For x = 236 days

`z=(x-\mu)/(\sigma/√(n) )=(236-247)/(16/√(20) )=-3.07`

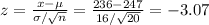
For x = 258 days

`z=(x-\mu)/(\sigma/√(n) )=(258-247)/(16/√(20) )=3.07`

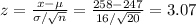
From the normal distribution table, P(236 < x < 258) = P(-3.07 < z < 3.07) = P(z < 3.07) - P(z < -3.07) =0.9985 - 0.0011 = 0.9939