Answer:
The probability that at least two of the customers exceed their limit is 0.2642.
Explanation:
We are given that Past records indicate that the probability of customers exceeding their credit limit is 0.05.
On a given day, 20 customers place orders.
Let X = the number of customers who exceed their credit limit
The above situation can be represented through binomial distribution;
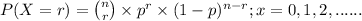
where, n = number of trials (samples) taken = 20 customers
r = number of success = at least two
p = probability of success which in our question is the probability
of customers exceeding their credit limit, i.e; 0.05.
So, X ~ Binom(n = 20, p = 0.05)
Now, the probability that at least two of the customers exceed their limit is given by = P(X
 2)
2)
P(X
 2) = 1 - P(X < 2) = 1 - P(X = 0) - P(X = 1)
2) = 1 - P(X < 2) = 1 - P(X = 0) - P(X = 1)
=

=
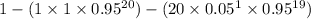
= 0.2642