Question

1. A rancher wants to build a rectangular pen, using one side of her barn for one side of the pen, and using 200m of fencing for the other three sides. What are the dimensions of the pen built this way that has the largest area?

Answer 1

100m by 50m.

Explanation:

Let the dimension of the rectangular pen be x and y

Area, A(x,y)=xy

Let the side opposite her barn = x

Since she wants to fence only three side

Perimeter = x+2y

Length of Fencing Available = 200m

Therefore:
`x+2y=200 \implies x=200-2y`

We want to maximize the area of the pen, A(x,y).

Substituting x=200-2y into A(x,y)=xy

`A(y)=y(200-2y)\\A(y)=200y-2y^2`

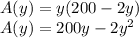
To maximize A(y), we find its derivative and solve for the critical points.

`A'(y)=200-4y\\$Setting $A'(y)=0\\200-4y=0\\200=4y\\y=50`

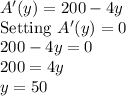
To ensure that this is a maximum, we use the second derivative test.

`A''(y)=-4`

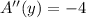
This is negative and thus, y=50 is a maximum point.

Recall:

x=200-2y

x=200-2(50)

x=200-100

x=100m

Therefore, the dimensions of the pen that has the largest area are 100m by 50m.