Answer:
![(d)/(dx)[f(x)+g(x)+h(x)] = \frac{9\cdot x^(8)}{\sqrt{1-x^(18)}} - 81\cdot x^(80)-2\cdot x](https://img.qammunity.org/2021/formulas/mathematics/college/5a2ij4iti1mnzszgllagsr8s71h9yutj27.png)
Explanation:
This derivative consist in the sum of three functions:
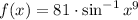 ,
,
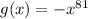 and
and
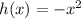 . According to differentiation rules, the derivative of a sum of functions is the same as the sum of the derivatives of each function. That is:
. According to differentiation rules, the derivative of a sum of functions is the same as the sum of the derivatives of each function. That is:
![(d)/(dx) [f(x)+g(x) + h(x)] = (d)/(dx) [f(x)]+(d)/(dx) [g(x)] +(d)/(dx) [h(x)]](https://img.qammunity.org/2021/formulas/mathematics/college/2kpwybj2wkcencfeq7z35qxg00j1ec3k2r.png)
Now, each derivative is found by applying the derivative rules when appropriate:
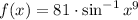 Given
Given
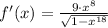 (Derivative of a arcsine function/Chain rule)
(Derivative of a arcsine function/Chain rule)
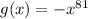 Given
Given
 (Derivative of a power function)
(Derivative of a power function)
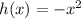 Given
Given
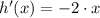 (Derivative of a power function)
(Derivative of a power function)
![(d)/(dx)[f(x)+g(x)+h(x)] = \frac{9\cdot x^(8)}{\sqrt{1-x^(18)}} - 81\cdot x^(80)-2\cdot x](https://img.qammunity.org/2021/formulas/mathematics/college/5a2ij4iti1mnzszgllagsr8s71h9yutj27.png) (Derivative for a sum of functions/Result)
(Derivative for a sum of functions/Result)