Final answer:
The volume of the cone is approximately 1,896.48 cubic meters.
Step-by-step explanation:
The volume of a cone can be calculated using the formula,
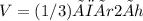 where r is the radius and h is the height of the cone.
where r is the radius and h is the height of the cone.
In this case, the diameter of the cone is given as 18 meters, which means the radius (r) will be half of that, i.e. 9 meters. The height (h) is given as 20 meters.
Substituting these values into the formula, we get
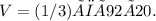
Using the value of π as 3.142, the volume of the cone is approximately 1,896.48 cubic meters.