Question

Greg is designing a clock face. Using the center of the clock face as the origin, he keeps its diameter at 10 units. Match the positions of the hours on the clock face to their corresponding coordinates. 3 o'clock (0, -5) 6 o'clock (5, 0) 9 o'clock (0, 5) 12 o'clock (-5, 0)

Answer 1

12 o'clock (0,5)

3 o'clock (5,0)

6 o'clock (0,-5)

9 o'clock (-5, 0)

Explanation:

Answer 2

3 o'clock (5, 0)

6 o'clock (0, -5)

9 o'clock (-5, 0)

12 o'clock (0, 5)

Explanation:

Given that diameter of clock = 10 units

We know that:

`Radius =(Diameter)/(2)`

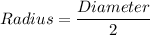
Radius = 5 units

The length of face will be equal to radius of clock i.e.

Length of face = 5 units

For the question, we need to visualize the clock time 3 o'clock, 6 o'clock, 9 o'clock and 12 o'clock on the coordinate xy axis.

Please refer to the attached graph to have a better understanding.

In every 3 hours the face will move
`90^\circ` i.e. one quadrant as per the location of clock faces.

At 3 o'clock the face will be on the positive x axis.

So coordinate (5, 0)

At 6 o'clock the face will be on the negative y axis.

So coordinate (0, -5)

At 9 o'clock the face will be on the negative x axis.

So coordinate (-5, 0)

At 12 o'clock the face will be on the positive y axis.

So coordinate (0, 5)

So, the answer is:

3 o'clock (5, 0)

6 o'clock (0, -5)

9 o'clock (-5, 0)

12 o'clock (0, 5)