Answer:
The claim made by Mar's is correct.
Explanation:
In this case we need to test whether M&M Mars Co.'s claim about the proportion of different colored M&Ms in a small bag is correct or not.
The hypothesis is:
H₀: The claim made by Mar's is not correct.
Hₐ: The claim made by Mar's is correct.
The proportions claimed are as follows:
Orange: 20%
Green: 16%
Blue: 24%
Other: 40%
A sample of 35 M&M's are selected.
The observed frequencies are:
Observed = {O = 4, G = 8, B = 5 and Ot = 18}
Compute the expected frequencies as follows:
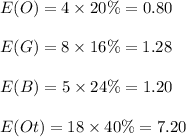
Compute the Chi-square statistic as follows:
![\chi^(2)=\sum[((O-E)^(2)))/(E)]](https://img.qammunity.org/2021/formulas/mathematics/high-school/hegs83o28k125stui15clvkpqhl0rmp8wd.png)
![=[((4-0.80)^(2))/(0.80)]+[((8-1.28)^(2))/(1.28)]+[((5-1.20)^(2))/(1.20)]+[((18-7.20)^(2))/(7.20)]\\\\=12.80+35.28+12.03+16.20\\\\=76.31](https://img.qammunity.org/2021/formulas/mathematics/high-school/3gyfpzy5ysczkme3sbtgqd51w0itkzn7h6.png)
The Chi-square test statistic value is 76.31.
The degrees of freedom of the test is:
df = n - 1
= 4 - 1
= 3
Compute the p-value as follows:
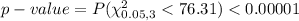
The p-value < 0.05.
The null hypothesis will be rejected at 5% level of significance.
Thus, concluding that the claim made by Mar's is correct.