Question

H

The coordinates of the vertices for the figure HIJK are
H(0,5), (3, 3), J(4, -1), and K(1, 1).
4
1
3
2
To determine if it is a parallelogram, use the converse
of the parallelogram diagonal theorem. This states that
if the diagonals
then the
quadrilateral is a parallelogram.
1
K
-5 4 3 2
-1
2
-1
The midpoint of HJ is
is (2, 2).
and the midpoint of IK
J
2
-4
Therefore, HIJK is a parallelogram because the
diagonals
which means
they bisect each other.
-5​

Answer 1

1 bisect each other

2 (2, 2)

3 have the same midpoint

Explanation:

Answer 2

The correct options are;

To determine if it is a parallelogram use the converse of the parallelogram diagram diagonal theorem. This states that if the diagonals bisect each other, then the quadrilateral is a parallelogram

The midpoint of
`\overline {HJ}` is (2, 2) and the midpoint of
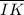`\overline {IK}` is (2, 2)

Therefore, HIJK is a parallelogram because the diagonals have the same (share a common) midpoint, which means they bisect each other

Explanation:

The given coordinates are;

H(0, 5), I(3, 3), J(4, -1), K(1, 1)

The diagonals are;

HJ and IK

The equations of the diagonals are;

Slope of segment HJ = (5 - (-1))/(0 - 4) = -3/2

Therefore, y - 5 = -3/2(x - 0)

When x = 0, y = 5, therefore c = 0 which gives;

y = -3/2x + 5

The slope of segment IK = (3 - 1)/(3 - 1) = 1

Therefore, y - 3 = x - 3

y = x

To get the intersection point, we have;

-3/2x + 5 = x

5 = 5/2x

x = 5/(5/2) = 2

y = x = 2

The coordinate of the intersection point is (2, 2)

The coordinates of the mid point if gotten from ((x₁ + x₂)/2, (y₁ + y₂)/2)

For HJ, we have;

((0 + 4)/2, (5 + (-1))/2) = (2, 2)

The coordinate of the midpoint of IK is ((3 + 1)2, (3 + 1)/2) = (2, 2)

Therefore, the diagonals bisect each other

Therefore we have;

To determine if it is a parallelogram use the converse of the parallelogram diagram diagonal theorem . This states that if the diagonals bisect each other, then the quadrilateral is a parallelogram

The midpoint of
`\overline {HJ}` is (2, 2) and the midpoint of
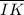`\overline {IK}` is (2, 2)

Therefore, HIJK is a parallelogram because the diagonals have a common midpoint which means they bisect each other