Question

In how many arrangements can 3 boys and 4 girls stand in a row such that no two boys are together?

Answer 1

Answer: 1440

Explanation:

To arrange 3 boys and 4 girls such that no two boys are together.

Since boys should be arranged between the girls.

So first arrange the girls.

Assume that the girls are placed, then there will be 5 spaces left for 3 boys.

The number of combinations to fill these places =
`^5C_3=(5!)/(3!2!)=(5*4)/(2)=10`

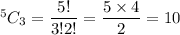
Also, 3 boys can arrange themselves in 3! =3 x 2 x 1 = 6 ways

4 girls can arrange themselves in 4! = 4x 3 x 2 x 1 = 24 ways

Then, the total number of arrangements = 10 x 6 x 24 = 1440

Hence, the required number of arrangements = 1440