Question

A three-point transverse bending test is conducted on a cylindrical specimen of aluminum oxide having a reported flexural strength of 390 MPa (56,600 psi). If the specimen radius is 2.5 mm (0.10 in.) and the support point separation distance is 30 mm (1.2 in.), predict whether or not you would expect the specimen to fracture when a load of 620 N (140 lbf) is applied. Calculate the value of flexural strength for this test.

Answer 1

Step-by-step explanation:

From the information given:

Flexural strength of the cylindrical specimen
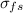`\sigma _(fs)` = 390 MPa (56,600 psi)

Radius of the cylindrical specimen R = 2.5 mm (0.10 in.)

the support point separation distance L = 30 mm (1.2 in.)

Load of Fracture
`F_f` = 620 N (140 lbf)

The flexural strength can be represented by the formula:

`\sigma f_s = (F_f L)/(\pi R^3)`

`\sigma f_s = (620 * (30 * 10 ^(-3)))/(\pi (2.5 * 10 ^(-3))^3)`

`\sigma f_s = (18.6)/(\pi * 1.5625 * 10^(-8))`

`\sigma f_s = 378916088.5`

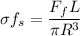
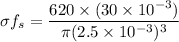
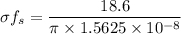
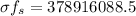
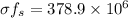`\sigma f_s = 378.9 * 10^(6)` N/m²

`\sigma f_s = 378.9 \ MPa`

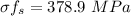
Therefore ; since that the derived stress is less than the flexural strength , It implies that the fracture might not occur.

We can therefore conclude that as the the derived stress is closer to the flexural strength (i.e the specimen), the prediction cannot be 100% certain because of the differences in the fluctuation of strength due to other combined factors such as the range where the specimen is being carried out, the temperature. etc