Answer:
(a)
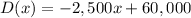
(b)

(c) x=12
(d)Optimal ticket price: $12
Maximum Revenue:$360,000
Explanation:
The stadium holds up to 50,000 spectators.
When ticket prices were set at $12, the average attendance was 30,000.
When the ticket prices were on sale for $10, the average attendance was 35,000.
(a)The number of people that will buy tickets when they are priced at x dollars per ticket = D(x)
Since D(x) is a linear function of the form y=mx+b, we first find the slope using the points (12,30000) and (10,35000).
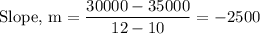
Therefore, we have:
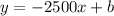
At point (12,30000)
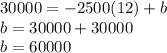
Therefore:
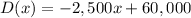
(b)Revenue
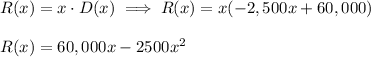
(c)To find the critical values for R(x), we take the derivative and solve by setting it equal to zero.
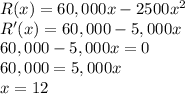
The critical value of R(x) is x=12.
(d)If the possible range of ticket prices (in dollars) is given by the interval [1,24]
Using the closed interval method, we evaluate R(x) at x=1, 12 and 24.
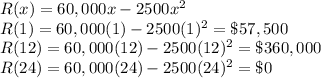
Therefore:
- Optimal ticket price:$12
- Maximum Revenue:$360,000