Question

A rectangular beam having b=300 mm and d=575 mm, spans 5.5 m face to face of simple supports. It is reinforced for flexure with 4φ32 bars that continue uninterrupted to the ends of the span. It is to carry a service dead load wD=30 kN/m (including self-weight) and a service live load =45 kN/m both uniformly distributed along the span. Design the shear reinforcement using φ10 vertical U stirrups. Use the equation (a) for Vc. Material strengths are fc’=22 and fy=420 MPa

Answer 1

provide 180 mm spacing

Step-by-step explanation:

GIVEN DATA

rectangular beam: (b) = 300 mm, (d) = 575 mm

reinforced for flexure = 4Ф32 bars

WD = 30 kN /m, WL = 45 kN/m

Wu = 1.4 * 30 + 1.6 * 45 = 114 kN/m

i) concrete shear stress ( vc)

100 Ac / bd = (100 * u *
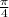`(\pi )/(4)` * 32^2) / 300 * 575 = 1.865

from table 3.8

when: 100 Ac / bd = 1.865 then Vc = 0.778 N/mm^2

Ultimate shear force = (114 *5.5) / 2 = 313.5 kN

design shear stress = V / bd = (313.5 * 10^3) / (300 * 575) = 1.82 N/mm^2

v < 0.8
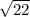`√(22)` = 1.82 < 3.75

design link provided according to

Asv / sv = b(v-vc) / 0.87 fy = 300(1.82 - 0.778) / 0.87 (420)

ASv / Sv = 0.855

From table 3.13 :the value of Asv / sv can be calculated as

`(0.855 - 0.785)/(0.897 - 0.785) = (x - 200)/(175 - 200)`

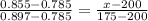
x = (-25) [ 0.625] + 200 = 184.375 mm

provide 180 mm spacing