Question

An object moves along a horizontal coordinate line in such a way that its position at time t is specified by s equals t cubed minus 3 t squared minus 24 t plus 8. Here s is measured in centimeters and t in seconds. When is the object slowing​ down; that​ is, when is its speed​ decreasing?

Answer 1

a)

The object slowing down S = -72 centimetres after t = 4 seconds

b)

The speed is decreasing at t = -2 seconds

The objective function S = 36 centimetres

Explanation:

Step(i):-

Given S = t³ - 3 t² - 24 t + 8 ...(i)

Differentiating equation (i) with respective to 'x'

`(dS)/(dt) = 3 t^(2) - 3 (2 t) - 24`

Equating Zero

3 t ² - 6 t - 24 = 0

⇒ t² - 2 t - 8 = 0

⇒ t² - 4 t + 2 t - 8 = 0

⇒ t (t-4) + 2 (t -4) =0

⇒ ( t + 2) ( t -4) =0

⇒ t = -2 and t = 4

Again differentiating with respective to 'x'

`(d^(2) S)/(dt^(2) ) = 6 t - 6`

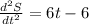
Step(ii):-

Case(i):-

Put t= -2

`(d^(2) S)/(dt^(2) ) = 6 t - 6 = 6 ( -2) -6 = -12 -6 = -18 <0`

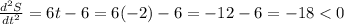
The maximum object

S = t³ - 3 t² - 24 t + 8

S = ( -2)³ - 3 (-2)² -24(-2) +8

S = -8-3(4) +48 +8

S = - 8 - 12 + 56

S = - 20 +56

S = 36

Case(ii):-

put t = 4

`(d^(2) S)/(dt^(2) ) = 6 t - 6 = 6 ( 4) -6 = 24 -6 = 18 >0`

The object slowing down at t =4 seconds

The minimum objective function

S = t³ - 3 t² - 24 t + 8

S = ( 4)³ - 3 (4)² -24(4) +8

S = 64 -48 - 96 +8

S = - 72

The object slowing down S = -72 centimetres after t = 4 seconds

Final answer:-

The object slowing down S = -72 centimetres after t = 4 seconds

The speed is decreasing at t = -2 seconds

The objective function S = 36 centimetres