Question

Lucy offers to play the following game with Charlie: "Let us show dimes to each other, each choosing either heads or tails. If we both show heads, I pay you $3. If we both show tails, I pay you $1. If the two don’t match, you pay me $2." Charlie reasons as follows. "The probability of both heads is 1/4, in which case I get $3. The probability of both tails is 1/4, in which case I get $1. The probability of no match is 1/2, and in that case I pay $2. So it is a fair game." Is he right? If not, (a) why not, and (b) what is Lucy’s expected profit from the game?

Answer 1

(a)Charlie is right

(b)$0

Explanation:

(a)A game is said to be a fair game when the probability of winning is equal to the probability of losing. Mathematically, a game is said to be fair when the expected value is zero.

In the game, the possible outcomes are: HH, HT, TH and TT.

Charlie wins when the outcome is HH, TT

• P(Charlie Wins)=2/4
• P(Charlie Losses)=2/4

Lucy wins when the outcome is HT or TH

• P(Lucy Wins)=2/4
• P(Lucy Losses)=2/4

Therefore, the game is fair. Charlie is right.

(b)

If the outcome is HH, Lucy pays $3.

If the outcome is HT or TH, Lucy gets $2.

If the outcome is TT, Lucy pays $1.

The probability distribution of Lucy's profit is given below:

`\left|\begin{array}c$Profit(x)&-\$3&-\$1&\$2\\P(x)&1/4&1/4&2/4\end{array}\right|`

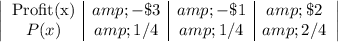
Expected Profit

`=(-3 * \frac14)+(-1* \frac14)+(2 * \frac24)\\=$0`

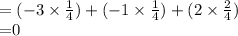
Lucy's expected profit from the game is $0.