Question

3. Maria is a veterinarian. She wants to know how the weight of a puppy is related to its length. To find out, Maria randomly selected 10 puppies that are two months old. She recorded the length and weight of each puppy in the table below. Part A. The data from the table are shown on the scatterplot. Draw an estimated line of best fit through the data points. (3 points) Part B. Use the scatterplot to answer these questions. a. What kind of correlation exists between the length and weight of the puppies? Explain. (2 points) b. Identify two points on the line of best fit that you drew in Part A. Use the two points to find the equation of the line. Write the equation of the best fit line in slope-intercept form. Show your work. (4 points: 1 point for identifying the coordinates of two points, 1 point for slope, 1 point for b-value, and 1 point for showing work)

Answer 1

Part A. I chose points (7,1.3) and (48,9.8)

Part B. a. Positive correlation; b. y = 0.21x - 0.2

Explanation:

Part A.

I chose the first and last points on the line — (7 in, 1.3 lb) and (48 in, 9.8 lb).

That put three points on the line, three above it, and four below.

Part B

a. Type of correlation

There is a positive correlation between the length of a puppy and its weight.

You would expect a longer dog to be bigger and weigh more than a shorter dog.

b. The equation for the line of best fit

The slope-intercept equation for a straight line is

y = mx + b

where m is the slope of the line and b is the y-intercept.

The line passes through the points (7,1.3) and (48, 9.8).

(i) Calculate the slope of the line

\begin{array}{rcl}

`m & = & (y_(2) - y_(1))/(x_(2) - x_(1))\\\\ & = & (9.8 - 1.3)/(48-9)\\\\& = & (8.5)/(41)\\\\& = & \textbf{0.21 lb/in}\\\\\end{array}`

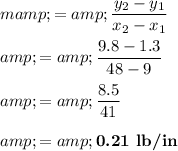
The slope of the line is 0.21 lb/in.

(ii) Locate the y-intercept

Put the slope and the coordinates of one point into the slope-intercept formula.

`\begin{array}{rcl}y & = & mx + b\\1.3 & = & 0.21*7 + b\\1.3 & = & 1.47 + b\\b & = & -0.2\\\end{array}`

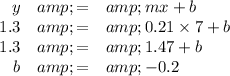
The y-intercept is at (0,-0.2)

(iii) Write the equation for the line

y = 0.21x - 0.2