Question

. Find the sum of the first 30 terms of the series:
16 + 19 + 22 +......​

Answer 1

1785

Explanation:

16+19+22+25+28+31+34+37+40+43+46+49+52+55+58+61+64+67+70+73+76+79+82+85+88+91+94+97+100+103=1785

Answer 2

The answer is 1785

Explanation:

The above sequence is an arithmetic sequence

The sum of an nth term of an arithmetic sequence is written as

`(n)/(2) (2a + (n - 1)d)`

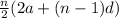
Where n is the number of terms

a is the first term

d is the common difference

From the question

n is 30

a is 16

d = 19 - 16 = 3

So the first 30 terms of the series is

`(30)/(2) (2(16) + (30 - 1) * 3) \\ = 15(32 + 29 * 3) \\ = 15(32 + 87) \\ = 15(119) \\ = 1785`

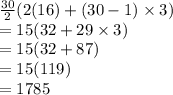
Hope this helps you.