Answer:
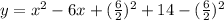
And solving we have:
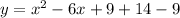
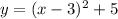
And we can write the expression like this:

The vertex for this case would be:
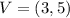
And the minimum for the function would be 3 and there is no maximum value for the function
Explanation:
For this case we have the following equation given:
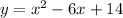
We can complete the square like this:
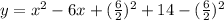
And solving we have:
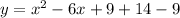
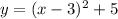
And we can write the expression like this:

The vertex for this case would be:
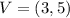
And the minimum for the function would be 3 and there is no maximum value for the function