Answer:
The correct option is;
C. 0.3337
Explanation:
We note that for a binomial probability distribution, we have;
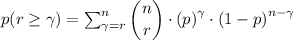
Which gives;
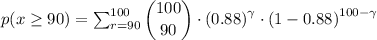

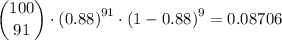

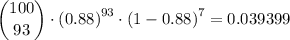
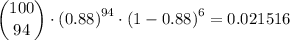
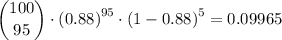
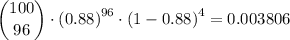
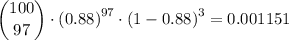
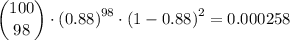
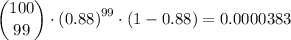
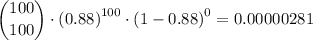
P(r≥90) is therefore 0.1083 + 0.08706 + 0.062456 + 0.039399 + 0.021516 + 0.09965 + 0.003806 + 0.001151 + 0.000258 + 0.0000383 + 0.00000281 = 0.333685 ≈ 0.3337.