Question

25 pts Must hv explanation The equation cos (35 degree) equals StartFraction a Over 25 EndFraction can be used to find the length of Line segment B C. What is the length of Line segment B C? Round to the nearest tenth. 14.3 in. 20.5 in. 21.3 in. 22.6 in.

Answer 1

A. 20.5 In

Explanation:

hello there, in order to solve a trigonometry solution you must know the law of cos sin rule..

please remember this formula...

• SOH
• CAH
• TOA

1. SOH.. Sin Ø =

`\sin(x) = (opposite)/( hypotenus)`

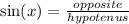
2. CAH..

`\cos(x) = (adjacemt)/(hypotenus)`

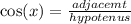
3. TOA

`\tan(x) = (opposite)/(adjacent)`

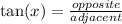
Based on the question.. the value is given such as

ø=35°

hypotenus = 25 inch

find the BC which is the adjacent..

so we have the value for hypotenus and the angle.. the only relationship that suits this category is CAH .

FORMULA FOR CAH

COS Ø = ADJACENT/ HYPOTENUS

then now we substitute the value given

`\cos(35) = (bc)/(25)`

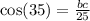
bring up the 25 to cos 35..

`25 \cos(35) = bc`

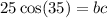
calculate the value of BC

`bc = 25 \cos(35)`

`bc = 20.47`

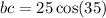
so the length of BC is equals to 20.47 or 20.5 In

Answer 2

a= 20.5in.

Explanation:

Using the law of cosine to solve the problem (adjacent/hypotenuse) you set up the equation cos(35)=a/25 since a is adjacent to the angle and since 25 is the hypotenuse. You then wanna multiply both sides of the equation by 25 to since you are dividing by 25 because opposites cancel out and you want to get the variable x alone and on one side. After doing this you get 25*cos(35)=x. You put this in a calculator and get 20.4788011072 and when you round it to the nearest tenth you get 20.5in.

Hope this helps :)