Question

est the hypothesis using the​ P-value approach. Be sure to verify the requirements of the test. Upper H 0 : p equals 0.89 versus Upper H 1 : p not equals 0.89 n equals 500 comma x equals 430 comma alpha equals 0.01 Is np 0 (1 minus p 0 )greater than or equals 10​? Select the correct choice below and fill in the answer box to complete your choice. ​(Type an integer or a decimal. Do not​ round.) A. ​No, because np 0 (1 minus p 0 )equals nothing. B. ​Yes, because np 0 (1 minus p 0 )equals 48.95. Your answer is not correct. Now find ModifyingAbove p with caret.

Answer 1

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the population proportion significantly differs from 0.89.

The requirements for the test are satisfief.

n(1-p)=70>10

Explanation:

This is a hypothesis test for a proportion.

There are 3 requirements to have a valid test of proportion: random sample, independence and normal.

For the first two (random and independent sample) we don't have details, but we assume the sampling has been random.

The latter can be verified by calculating np and n(1-p):

`np=430>10\\\\n(1-p)=70>10`

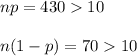
Both are bigger than 10, so the normal approximation can be considered appropiate.

The claim is that the population proportion significantly differs from 0.89.

Then, the null and alternative hypothesis are:

`H_0: \pi=0.89\\\\H_a:\pi\\eq 0.89`

The significance level is 0.01.

The sample has a size n=500.

The sample proportion is p=0.86.

`p=X/n=430/500=0.86`

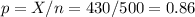
The standard error of the proportion is:

`\sigma_p=\sqrt{(\pi(1-\pi))/(n)}=\sqrt{(0.89*0.11)/(500)}\\\\\\ \sigma_p=√(0.000196)=0.014`

Then, we can calculate the z-statistic as:

`z=(p-\pi+0.5/n)/(\sigma_p)=(0.86-0.89+0.5/500)/(0.014)=(-0.029)/(0.014)=-2.072`

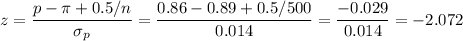
This test is a two-tailed test, so the P-value for this test is calculated as:

`\text{P-value}=2\cdot P(z<-2.072)=0.038`

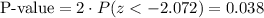
As the P-value (0.038) is greater than the significance level (0.01), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the population proportion significantly differs from 0.89.