Answer:
The final velocity of the 30 g object is 16.8 cm/s
The final velocity of the 13 g object is 21.3 cm/s
Step-by-step explanation:
Let's study the elastic collision with conservation of linear momentum, assigning object 1 to the 30 g object, and object 2 to the 13 gr object:
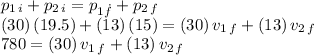
so we can write one of the unknowns in terms of the other one:
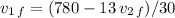
Now we analyze the equation for conservation of kinetic energy that verifies in elastic collisions:
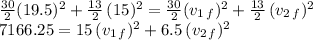
now we can write this quadratic equation replacing
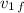 with its expression in terms of
with its expression in terms of
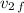 and solve it (with the help of a graphing calculator is simpler by looking for the roots).
and solve it (with the help of a graphing calculator is simpler by looking for the roots).
We get two answers for
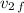 : one 15 cm/s, and the other one 21.28 cm/s.
: one 15 cm/s, and the other one 21.28 cm/s.
We select the 21.28 cm/s answer since otherwise, the situation is the same as the initial one at which the second object was moving at 15 cm/s.
This velocity can be rounded to one decimal to: 21.3 cm/s
Given the value 21,28 for
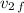 , then:
, then:
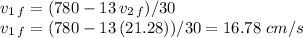
which can be rounded to 16.8 cm/s