Question

a normal population has a mean 100 and variance 25. how large must the sample size be if we want the standard error of the sample average to be at most 1.5

Answer 1

A sample size of 12 is needed.

Explanation:

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation(square root of the variance)
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation, which is also called standard error,
`s = (\sigma)/(√(n))`.

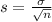In this question:

`\sigma = √(25) = 5`

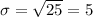
How large must the sample size be if we want the standard error of the sample average to be at most 1.5

We need n for which s = 1.5.

`s = (\sigma)/(√(n))`

`1.5 = (5)/(√(n))`

`1.5√(n) = 5`

`√(n) = (5)/(1.5)`

`(√(n))^(2) = ((5)/(1.5))^(2)`

`n = 11.11`

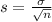
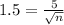
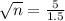
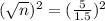
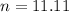
Rounding up

A sample size of 12 is needed.