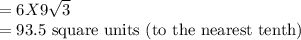Question

Can anyone please explain? Need some help :)

A regular hexagon is inscribed in a circle with a diameter of 12 units. Find the area of the hexagon. Round your answer to the nearest tenth. (there's no picture included)

Answer 1

93.5 square units

Explanation:

Diameter of the Circle = 12 Units

Therefore:

Radius of the Circle = 12/2 =6 Units

Since the hexagon is regular, it consists of 6 equilateral triangles of side length 6 units.

Area of the Hexagon = 6 X Area of one equilateral triangle

Area of an equilateral triangle of side length s =
`(√(3) )/(4)s^2`

Side Length, s=6 Units

`\text{Therefore, the area of one equilateral triangle =}(√(3) )/(4)* 6^2\\\\=9√(3) $ square units`

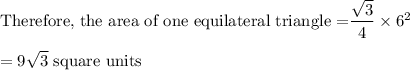
Area of the Hexagon

`= 6 X 9√(3) \\=93.5$ square units (to the nearest tenth)`