Complete question:
A School of Public Health completed a study on alcohol consumption on college campuses. They concluded that 20.1?% of women attending? all-women colleges abstained from? alcohol, compared to 17.1?% of women attending coeducational colleges. Approximately 5.2?% of women college students attend? all-women schools. Complete parts? (a) and? (b) below.
a) what is the probability that a randomly selected female student abstains from alcohol
b) If a randomly selected female student abstains from alcohol, what is the probability she attends a coeducational college?
Answer:
a) 0.173
b) 0.94
Explanation:
Given:
Probability of women attending all women colleges, probabilty of abstained from alcohol P(Ab|AW) = 0.201
Probability of women attending coed colleges, probabilty of abstained from alcohol P(Ab|CE) =0.171
Let probability of women attending all women college, P(AW) =0.052
Let probability of women attending coed, P(CE) = 1 - 0.052 =0.948
a) To find the probability that a randomly selected female student abstains from alcohol, use the formula below:
P(Ab) =P(Ab|AW)*P(AW)+P(Ab|CE)*P(CE)
=(0.201 * 0.052) + (0.171 * 0.948) = 0.17256
Probability that a randomly selected female student abstains from alcohol is 0.17256 ≈ 0.173
b) If randomly selected female student abstains from alcohol, probability she attends a coeducational college. Use the formula below:
P(CE|Ab) =P(Ab|CE)*P(CE)/P(Ab) =
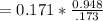
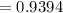
≈ 0.94