Question

The completion times for a job task range from 11.1 minutes to 19.2 minutes and are thought to be uniformly distributed. What is the probability that it will require between 14.8 and 16.5 minutes to perform the task?

Answer 1

`P(14.8< X<16.5)= (16.5-11.1)/(19.2-11.1) -(14.8-11.1)/(19.2-11.1)= 0.667-0.457= 0.210`

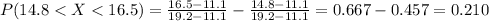
The probability that it will require between 14.8 and 16.5 minutes to perform the task is 0.210

Explanation:

Let X the random variable "completion times for a job task" , and we know that the distribution for X is given by:

`X \sim Unif (a= 11.1, b= 19.2)`

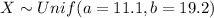
And for this case we wantto find the following probability:

`P(14.8< X<16.5)`

And for this case we can use the cumulative distribution given by:

`F(x) =(x-a)/(b-a) , a\leq X \leq b`

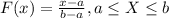
And using this formula we got:

`P(14.8< X<16.5)= (16.5-11.1)/(19.2-11.1) -(14.8-11.1)/(19.2-11.1)= 0.667-0.457= 0.210`

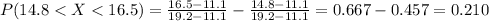
The probability that it will require between 14.8 and 16.5 minutes to perform the task is 0.210