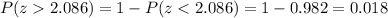Answer:
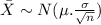
And we want to find the following probability:
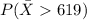
And we can use the z score formula given by:
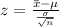
And replacing we got:
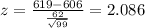
And we can use the normal standard distirbution and the complement rule to find the probability:
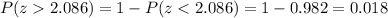
Explanation:
For this problem we have the following parameters given:
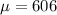 represent the mean
represent the mean
 represent the true deviation
represent the true deviation
 represent the sample size
represent the sample size
For this case since the sample size is >30 we can use the central limit theorem and we can u se the following distribution for the sample mean
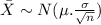
And we want to find the following probability:
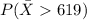
And we can use the z score formula given by:
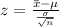
And replacing we got:
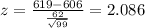
And we can use the normal standard distirbution and the complement rule to find the probability: