Answer:
(A)The pool is 1 meter deep.
Explanation:
The graph that is to be interpreted is attached below.
From the graph:
We have a bold red line at y=-1
Since above the line is shaded(in red), the first inequality is: y ≥ -1
Next
The blue line is dotted and passes through points (0, 0) and (-2, -1).
We determine the equation of the blue line, y=mx+b.
Slope of the blue line,
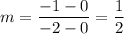
Since the y-intercept, b=0
The equation of the line is:
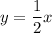
Since below the blue line is shaded, the inequality showing the shaded area is:
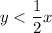
Therefore, a solution to the set of inequalities is (2, -1)
Since the pool is 1 meter deep and anything beyond this is outside the feasible region, the constraint is:
(A). The pool is 1 meter deep.