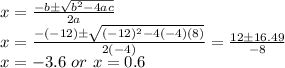Question

Equation 3 should represent a parabola that is a vertical stretch of the parent function and has a y-intercept greater than 3 and opens down. Equation 3:___________________________________________________________________ What strategy are you using to solve this equation and why? ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ ______________________________________________________________________________ Show your work and solution for solving this equation:

Answer 1

The answer is given below

Explanation:

The equation of a quadratic function is given by:

Ax² + Bx + C where A, B and C are the coefficients of the quadratic equation. If the coefficient of x² (i.e A) is a negative value then the graph opens down, the value of C determines the y intercept.

The equation of a parabola with y-intercept greater than 3 and opens down can be given as -2x² -6x + 4. Since the coefficient of x² is negative it opens down and C = 4, it has a y intercept of 4 > 3

Let us assume a vertical stretch of 2, the new equation becomes:

2(-2x² -6x + 4)

-4x² - 12x + 8

To solve:

-4x² - 12x + 8 = 0

A = -4, B = -12 and C = 8

Using the quadratic formula:

`x=(-b \pm √(b^2-4ac) )/(2a)\\ x=(-(-12) \pm √((-12)^2-4(-4)(8)) )/(2(-4))=(12 \pm 16.49)/(-8) \\x= -3.6 \ or \ x=0.6`