Question

The area of the pond is approximately equal to the area of a circle with radius 297m. Find the mass of the ice. Answer in kilograms. g

Answer 1

mass of the ice is 254980463.8T kg

where T is the value of the thickness omitted in the question.

Step-by-step explanation:

The ice on Walden Pond is .......... thick. The area of the pond is approximately equal to the area of a circle with radius 297 m. Find the mass of the ice. Answer in kg.

The value of the thickness of the ice T is omitted, but I will show the solution, and the real answer can be gotten by multiplying the final calculated answer here by the thickness of the ice omitted.

Given the radius of the equivalent circle of the ice = 297 m'

the area of the ice can be gotten from area A =
`\pi r^(2)` =
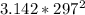`3.142*297^(2)` = 277152.678 m^2

recall that the density of ice p ≅ 920 kg/m^3

also,

density of ice p = (mass of ice, m) ÷ (volume of ice, v)

i.e p = m/v

and,

m = pv

substituting the value of the density of water p into the equation, we have,

mass of the ice, m = 920v ....... equ 1

The volume of the ice above will be = (area of the ice, A) x (thickness of the ice, T)

i.e v = AT

substituting the value of area A into the equation, we have

v = 277152.678T ......equ 2

substitute value of v into equ 1

mass of the ice, m = 920 x (277152.678T)

mass of the ice, m = 254980463.8T kg

where T is the thickness of the ice

NB: To get the mass, multiply this answer with the thickness T given in the question.