Answer:
A 95% confidence interval for this population proportion is [0.081, 0.159].
Explanation:
We are given that a market research company conducted a survey to find the level of affluence in a city.
Out of 267 persons who replied to their survey, 32 are considered affluent.
Firstly, the pivotal quantity for finding the confidence interval for the population proportion is given by;
P.Q. =
 ~ N(0,1)
~ N(0,1)
where,
 = sample proportion of people who are considered affluent =
= sample proportion of people who are considered affluent =
 = 0.12
= 0.12
n = sample of persons = 267
p = population proportion
Here for constructing a 95% confidence interval we have used One-sample z-test for proportions.
So, 95% confidence interval for the population proportion, p is ;
P(-1.96 < N(0,1) < 1.96) = 0.95 {As the critical value of z at 2.5% level
of significance are -1.96 & 1.96}
P(-1.96 <
 < 1.96) = 0.95
< 1.96) = 0.95
P(
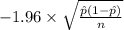 <
<
 <
<
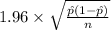 ) = 0.95
) = 0.95
P(
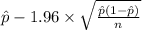 < p <
< p <
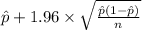 ) = 0.95
) = 0.95
95% confidence interval for p = [
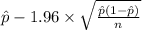 ,
,
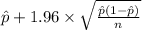 ]
]
= [
 ,
,
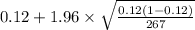 ]
]
= [0.081, 0.159]
Therefore, a 95% confidence interval for this population proportion is [0.081, 0.159].