Question

A clinical trial tests a method designed to increase the probability of conceiving a girl. In the study 290 babies were​ born, and 261 of them were girls. Use the sample data to construct a 99​% confidence interval estimate of the percentage of girls born. Based on the​ result, does the method appear to be​ effective?

Answer 1

The 99​% confidence interval estimate of the percentage of girls born is between 85.46% and 94.54%.

Usually, babies are equally as likely to be boys or girls. Here, it is desired to increase the probability of conceiving a girl, which is achieved, considering the lower bound of the confidence interval is considerably above 50%.

Explanation:

Confidence interval for the proportion:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

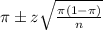
In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`.

For this problem, we have that:

`n = 290, \pi = (261)/(290) = 0.9`

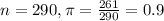
99% confidence level

So
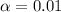`\alpha = 0.01`, z is the value of Z that has a pvalue of
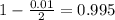`1 - (0.01)/(2) = 0.995`, so
`Z = 2.575`.

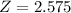The lower limit of this interval is:

`\pi - z\sqrt{(\pi(1-\pi))/(n)} = 0.9 - 2.575\sqrt{(0.9*0.1)/(290)} = 0.8546`

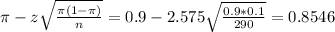
The upper limit of this interval is:

`\pi + z\sqrt{(\pi(1-\pi))/(n)} = 0.9 + 2.575\sqrt{(0.9*0.1)/(290)} = 0.9454`

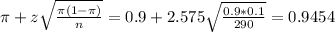
Percentage:

Proportion multplied by 100.

0.8546*100 = 85.46%

0.9454*100 = 94.54%

The 99​% confidence interval estimate of the percentage of girls born is between 85.46% and 94.54%.

Based on the​ result, does the method appear to be​ effective?

Usually, babies are equally as likely to be boys or girls. Here, it is desired to increase the probability of conceiving a girl, which is achieved, considering the lower bound of the confidence interval is considerably above 50%.