Question

Help me. The graph of a polynomial with unknown a is given. Write a polynomial

function of lowest possible degree for the graph. Leave your answer in
factored form

Answer 1

`f(x)=(7)/(20)\left(x+2\right)^(2)\left(x-1\right)`.

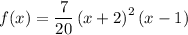Explanation:

If a graph touches the x-axis at x=c, then (x-c) is a factor of function.

From the given graph it is clear that the graph of function intersect x-axis at x=1 and touch the x-axis at x=-2.

It means (x-1) and (x+2) are not factors of given function but power of (x+2) must be 2.

So, the required function is

`f(x)=a(x+2)^2(x-1)` ...(1)

where, a is a constant.

From the given figure it is clear that the graph passes through the point (-4,-7). So, substitute x=-4 and f(x)=-7 in the above function.

`-7=a(-4+2)^2(-4-1)`

`-7=a(-2)^2(-5)`

`-7=-20a`

`(7)/(20)=a`

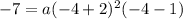
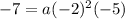
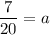
Substitute
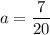`a=(7)/(20)` in (1).

`f(x)=(7)/(20)(x+2)^2(x-1)`

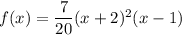
Therefore, the required function is
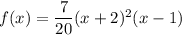`f(x)=(7)/(20)(x+2)^2(x-1)`.