Question

A professor would like to test the hypothesis that the average number of minutes that a student needs to complete a statistics exam has a standard deviation that is less than 5.0 minutes. A random sample of 15 students was selected and the sample standard deviation for the time needed to complete the exam was found to be 4.0 minutes. Using α = 0.05, the conclusion for this hypothesis test would be that because the test statistic is

Answer 1

`\chi^2 =(15-1)/(25) 16 =8.96`

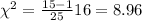
The degrees of freedom are given by:

`df = n-1 = 15-1=14`

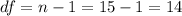
The p value for this case would be given by:

`p_v =P(\chi^2 <8.96)=0.166`

Since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true deviation is not ignificantly lower than 5 minutes

Explanation:

Information given

`n=15` represent the sample size

`\alpha=0.05` represent the confidence level

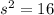`s^2 =16` represent the sample variance

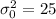`\sigma^2_0 =25` represent the value that we want to verify

System of hypothesis

We want to test if the true deviation for this case is lesss than 5minutes, so the system of hypothesis would be:

Null Hypothesis:
`\sigma^2 \geq 25`

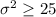
Alternative hypothesis:
`\sigma^2 <25`

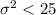
The statistic is given by:

`\chi^2 =(n-1)/(\sigma^2_0) s^2`

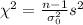
And replacing we got:

`\chi^2 =(15-1)/(25) 16 =8.96`

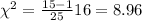
The degrees of freedom are given by:

`df = n-1 = 15-1=14`

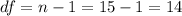
The p value for this case would be given by:

`p_v =P(\chi^2 <8.96)=0.166`

Since the p value is higher than the significance level we have enough evidence to FAIL to reject the null hypothesis and we can conclude that the true deviation is not ignificantly lower than 5 minutes