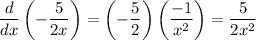Answer:
The constant factor remains a multiplier.
Explanation:
The derivative of "a·f(x)" is a·f'(x). That is, the constant multiplier remains a constant multiplier, unchanged.
For ax^-1, the derivative is a(-x^-2) per the power rule. For your value of a=-5/2, this is ...