Answer:
(12, 2) is the original point on the graph of
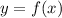 .
.
Explanation:
Given:
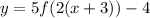 has a point (3, 6) on its graph.
has a point (3, 6) on its graph.
To find:
Original point on graph
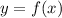 = ?.
= ?.
Solution:
We are given that The point (3, 6) is on the graph of
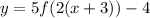
If we put x = 3 and y = 6 in
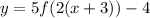 , it will satisfy the equation.
, it will satisfy the equation.
Let us the put the values and observe:
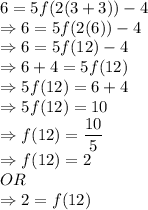
Now, let us compare the above with the following:
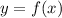
we get y = 2 and x = 12
So, the original point on graph of
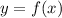 is (12, 2).
is (12, 2).