Question

Solve this rational equation:

Х
1
x – 4
+
=
2
x2 - 6x + 8
x – 2

Answer 1

Hey there! :)

Answer:

x = -1.

Explanation:

`(1)/(x-4)+ (x)/(x-2)= (2)/(x^(2)-6x+8 )`

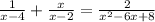
Make each fraction have a common denominator:

`(1(x-2))/(x^(2)-6x+8)+ (x(x-4))/(x^(2)-6x+8)= (2)/(x^(2)-6x+8 )`

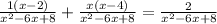
Simplify:

`(x-2)/(x^(2)-6x+8)+ (x^(2)-4x )/(x^(2)-6x+8)= (2)/(x^(2)-6x+8 )`

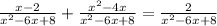
Disregard the denominator and solve the numerators:

x - 2 + x² - 4x = 2

Combine like terms:

x² - 3x - 2 = 2

x² - 3x - 4 = 0

Factor:

(x - 4)(x + 1)

***Only one of these solutions works because if x = 4, the denominator of the first fraction would be 0, which is undefined. Therefore, the only possible solution is x = -1.