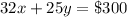Answer:
(a)
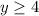
(b)

(c)
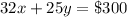
Explanation:
We are given that a pen costs 32 cents and a pencil costs 25 cents. Kwok buys x pens and y pencils.
We have to express each of the following statements as an inequality.
(a) Kwok buys at least 4 pencils;
As it is given that the number of pencils bought by Kwok is y pencils and our statement states that Kwok buys at least 4 pencils.
So, the inequality that represents the above situation is given by;
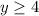
(b) Kwok buys more pens than pencils;
As it is given that the number of pens bought by Kwok is x pens and the number of pencils bought by Kwok is y pencils and our statement states that Kwok buys more pens than pencils.
So, the inequality that represents the above situation is given by;

(c) Kwok spends no more than 3.00 dollars;
As we know that a pen costs 32 cents and a pencil costs 25 cents.
Also, Expenditure = Price
 Quantity
Quantity
So, the inequality that represents the above situation is given by;