Answer:
n = 7
Explanation:
The sum of the interior angles of a polygon is 9
 . If x is 3 greater than the number of sides of the polygon, how many sides does the polygon have?
. If x is 3 greater than the number of sides of the polygon, how many sides does the polygon have?
The sum of the interior angles of a concave polygon can be found using the formula S = (n - 2)*180.
n= number of sides of the polygon
n-2 * 180 = the sum of the interior angles
9
 = the sum of the interior angles
= the sum of the interior angles
9
 = (n-2) *180
= (n-2) *180
x= 3+ n
9
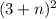 =(n-2) *180
=(n-2) *180
9 (9+6n+n^2) = (n-2) *180
81+54n+9n^2 = (n-2) *180
n = 7