Question

Which of these systems of linear equations has no solution? 2 x + 8 y = 15. 4 x + 16 y = 30. 2 x minus y = 18. 4 x + 2 y = 38. 4 x + 7 y = 17. 8 x minus 14 y = 36. 4 x minus 3 y = 16. 8 x minus 6 y = 34.

Answer 1

d

Explanation:

Answer 2

The correct answer is:

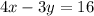`4 x -3 y = 16` and

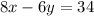`8 x -6 y = 34` are the equations that have no solution.

Explanation:

First of all, let us have a look at the rules for a pair of lines, that have solution or no solution.

Let the equations be:

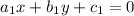`a_1x+b_1y+c_1=0` and

`a_2x+b_2y+c_2=0`

1. There exists exactly one solution:

`(a_1)/(a_2)\\eq(b_1)/(b_2)`

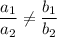
2. There exists infinitely many solutions i.e. lines are identical.

`(a_1)/(a_2)=(b_1)/(b_2)=(c_1)/(c_2)`

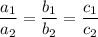
3. There exists No solutions i.e. lines are parallel and will never intersect.

`(a_1)/(a_2)=(b_1)/(b_2)\\eq(c_1)/(c_2)`

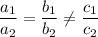
Now, let us have a look at the given pair of lines:

Option a)

`2 x + 8 y = 15\\4 x + 16 y = 30.`

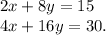
The ratio:

`(2)/(4)=(8)/(16)=(15)/(30) = (1)/(2)`

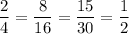
Hence, the lines are identical, infinite solutions.

Option b)

`2 x - y = 18\\4 x + 2 y = 38`

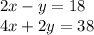
The ratio:

`(2)/(4)\\eq (-1)/(2)`

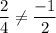
Hence, exactly one solution.

Option c)

`4 x + 7 y = 17\\8 x -14 y = 36`

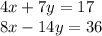
The ratio:

`(4)/(8)\\eq (-7)/(14)\\(1)/(2)\\eq (-1)/(2)`

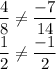
Hence, exactly one solution.

Option d)

`4 x - 3 y = 16\\8 x - 6 y = 34.`

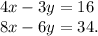
The ratio:

`(4)/(8)= (-3)/(-6)\\eq(16)/(34)\\\Rightarrow (1)/(2)= (1)/(2)\\eq(8)/(17)`

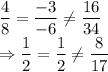
Hence, There is no solution.

The correct answer is:

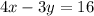`4 x -3 y = 16` and

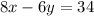`8 x -6 y = 34` are the equations that have no solution.