Question

7. The mean age at first marriage for respondents in a survey is 23.33,

with a standard deviation of 6.13. For an age at first marriage of 33.44,
the proportion of area beyond the Z score associated with this age is
.05. What is the percentile rank for this score?
​

Answer 1

`\mu = 23.33, \sigma =6.13`

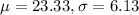
And for this case we are analyzing the value os 33.44 and we can use the z score formula given by:

`z=(X -\mu)/(\sigma)`

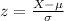
And replacing we got:

`z=(33.44 -23.33)/(6.13)= 1.649`

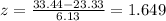
We know that the proportion of area beyond the Z score associated with this age is .05 so then the percentile would be: 95

Explanation:

For this case we have the following parameters:

`\mu = 23.33, \sigma =6.13`

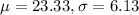
And for this case we are analyzing the value os 33.44 and we can use the z score formula given by:

`z=(X -\mu)/(\sigma)`

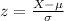
And replacing we got:

`z=(33.44 -23.33)/(6.13)= 1.649`

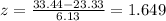
We know that the proportion of area beyond the Z score associated with this age is .05 so then the percentile would be: 95