Question

The potential energy function

U(x,y)=A[(1/x2) + (1/y2)] describes a conservative force, where A>0.
Derive an expression for the force in terms of unit vectors i and j.

Answer 1

`F=-2A[(1)/(x^3)\hat{i}+(1)/(y^3)\hat{j}]`

Step-by-step explanation:

You have the following potential energy function:

`U(x,y)=A[(1)/(x^2)+(1)/(y^2)}]` (1)

A > 0 constant

In order to find the force in terms of the unit vectors, you use the gradient of the potential function:

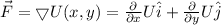`\vec{F}=\bigtriangledown U(x,y)=(\partial)/(\partial x)U\hat{i}+(\partial)/(\partial y)U\hat{j}` (2)

Then, you replace the expression (1) into the expression (2) and calculate the partial derivatives:

`\vec{F}=A(\partial)/(\partial x)[(1)/(x^2)+(1)/(y^2)]} \hat{i}+A(\partial)/(\partial x)[(1)/(x^2)+(1)/(y^2)]\hat{j}\\\\\vec{F}=A(-2x^(-3))\hat{i}+A(-2y^(-3))\hat{j}\\\\F=-2A[(1)/(x^3)\hat{i}+(1)/(y^3)\hat{j}]`(3)

The result obtained in (3) is the force expressed in terms of the unit vectors, for the potential energy function U(x,y).