Question

The equilibrium fraction of lattice sites that are vacant in electrum (a silver gold alloy) at 300K C is 9.93 x 10-8. There are 5.854 x 1015 vacancies/cm3.The atomic mass of electrum is 146.08 g/mol.Avagadro number 6.02210^23 atom/mol.Calculate the density of electrum in g/cm3.

Answer 1

the density of the electrum is 14.30 g/cm³

Step-by-step explanation:

Given that:

The equilibrium fraction of lattice sites that are vacant in electrum =
`9.93*10^(-8)`

Number of vacant atoms =
`5.854 * 10^(15) \ vacancies/cm^3`

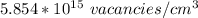
the atomic mass of the electrum = 146.08 g/mol

Avogadro's number =
`6.022*10^{23`

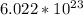
The Number of vacant atoms = Fraction of lattice sites × Total number of sites(N)

`5.854*10^(15)` =
`9.93*10^(-8)` × Total number of sites(N)

Total number of sites (N) =
`(5.854*10^(15))/(9.93*10^(-8))`

Total number of sites (N) =
`5.895*10^(22)`

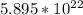
From the expression of the total number of sites; we can determine the density of the electrum;

`N = (N_A \rho _(electrum))/(A_(electrum))`

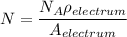
where ;

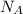`N_A` = Avogadro's Number

`\rho_(electrum) =` density of the electrum

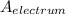`A_(electrum)` = Atomic mass

`5.895*10^(22) = (6.022*10^(23)* \rho _(electrum))/(146.08)`

`5.895*10^(22) *146.08}= 6.022*10^(23)* \rho _(electrum)`

`8.611416*10^(24)= 6.022*10^(23)* \rho _(electrum)`

`\rho _(electrum)=(8.611416*10^(24))/(6.022*10^(23))}`

`\mathbf{ \rho _(electrum)=14.30 \ g/cm^3}`

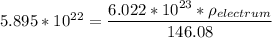
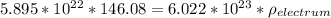
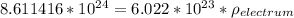
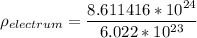
Thus; the density of the electrum is 14.30 g/cm³