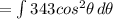Answer:
Explanation:
1. Given the integral function
 , using trigonometric substitution, the substitution that will be most helpful in this case is substituting x as
, using trigonometric substitution, the substitution that will be most helpful in this case is substituting x as
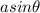 i.e
i.e
 .
.
All integrals in the form
 are always evaluated using the substitute given where 'a' is any constant.
are always evaluated using the substitute given where 'a' is any constant.
From the given integral,
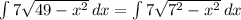 where a = 7 in this case.
where a = 7 in this case.
The substitute will therefore be
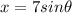
2.) Given
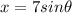
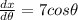
cross multiplying

3.) Rewriting the given integral using the substiution will result into;