Answer:
Lateral area of the pyramid = 120 square units
Explanation:
In the figure attached,
A pyramid has been given with square base with edges of 12 units and perpendicular height as 8 units.
Lateral area of a pyramid = Area of the lateral sides
Area of one lateral side =

=
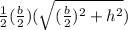 [Since l =
[Since l =
 ]
]
=

=
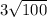
= 30 units²
Now lateral area of the pyramid = 4 × 30 = 120 square units